Founded by San Fransisco's Exploratorium (a public learning laboratory), π-day[1] is celebrated on March 14th every year, because it is the 3rd month of the year, and 14th day of that month, and in the mm/dd format this is written as 3/14, or 3.14, which is π correct to two decimal places (or three significant figures) — meaning, of course, that the day should be more accurately referred to as Pi-To-Two-Decimal-Places day, or Pi-To-Three-Significant-Figures day! UNESCO also proclamated the day in 2020 as the International Day of Mathematics. It is a day for schools to unashamedly revel in a pure celebration of π and, by association, mathematics.
I offer in this post therefore a few little somethings that teachers may wish to share with their students, a few little somethings moreover that teachers may want to delve deeper into and thus take further with their students, marking π-day in the spirit of celebration that it promotes. In addition, and in complement, schools and teachers may wish to carry out some Random Acts of Maths (download them here) over the course of the day, and perhaps hold a Favourite Number Election (find suggestions for use and download a ballot paper here).
- Archimedes' constant
- The origin of the symbol π for \(C/d\)
- π formulae
- π-day and other π birthdays
- Famous π-day birthdays
- The greatest π-day birthday in history
- π o'clock
- π-day is the 73rd day of the year
- π Primes
- Play the π-day lottery
- π as the Prime Counting Function
- Fake proofs for π
- Memorising π
- Continued Fractions for π
- Pilish (Self referential stories for π)
- The Music of π
- π Puns and Jokes
- π inspired Art
- Other links to film, audio, websites, and articles
- Notes and References
(Click here to return to the menu)
\[\frac{{223}}{{71}} < \pi < \frac{{22}}{7}\]
Or, in mixed numbers:
\[3\frac{{10}}{{71}} < \pi < 3\frac{1}{7}\]
Or, to six decimal places:
\[3.140845 < \pi < 3.\dot 14285\dot 7\]
This 'method of exhaustion' continued to be used for many years, until the invention of calculus and the use of arctangents allowed us to find π to ever increasing numbers of decimal places. π was found to 100 decimal places in such a way by John Machin, as published in William Jones' 1706 book Synopsis palmariorum matheseos). The Archimedean 'method of exhaustion' was used most notably by German mathematician Ludoph van Ceulen in the 16th Century, who reached a regular polygon with 4,611,686,018,427,387,904 sides to arrive at a value of π correct to 35 digits, calculated, of course, by hand.
Following Archimedes' approach with younger secondary students will enhance their appreciation of mathematics history, which in turn can positively influence their perception of mathematics, of what mathematics is, profoundly. For more on how the Archimedes' upper and lower bounds for π were found, see this video (17min) from Math Without Borders. With this interactive demonstration from the Wolfram Demonstrations Project, students can also explore how the upper and lower bounds for π change as the number of sides to the polygons change.
(Click here to return to the menu)
π was first used to denote the ratio of a circle's circumference to its diameter by Welsh mathematician William Jones in his 1706 book Synopsis palmariorum matheseos. In the same book, as mentioned above, π was shown to 100 decimal places, as 'computed', in Jones' words, 'by the Accurate and Ready Pen of the Truly Ingenious Mr. John Machin', using what has since become known as 'Machin's formula'. (See this this post for a little more on Jones' notation.) You can peruse the book electronically here, finding π (as C/d) for the first recorded time in history on p243, as extracted in the first image below, then more explicitly on p263, as extracted in the second image below.
(Click here to return to the menu)
Many awe-inspiring formulae have been found for π, in exact or approximate, iterative forms. Below is a small selection of some classics that may be interesting to explore with students, possibly comparing the relative accuracy of respective formulae and their relative rates of convergence.
Viète's formula. François Viète published in 1593 what is commonly accepted to be the first instance of an infinite product known in mathematics. It is derived by comparing the areas of regular polygons with 2n and 2n + 1 sides inscribed in a circle, and converges to π fairly rapidly. (Play with Viète's formula with this interactive Wolfram demonstration.)
Wallis' product. John Wallis published this formula in his 1656 Arithmetica Infinitorum. Recently, Wallis' product was 'discovered,' quite incredibly, 'hidden in [the] hydrogen atom', as shared with the world through this paper — 'Quantum mechanical derivation of the Wallis formula for pi' — from the Journal of mathematical Physics, revealing a hitherto unknown and beautiful connection between mathematics and Physics. (Visualise Wallis' sieve approximation for π with this interactive Wolfram demonstration and watch this typically wonderful video from Grant Sanderson providing a 'new proof of the Wallis formula'.)
Many awe-inspiring formulae have been found for π, in exact or approximate, iterative forms. Below is a small selection of some classics that may be interesting to explore with students, possibly comparing the relative accuracy of respective formulae and their relative rates of convergence.
\[\pi = 2 \times \frac{2}{{\sqrt 2 }} \times \frac{2}{{\sqrt {2 + \sqrt 2 } }} \times \frac{2}{{\sqrt {2 + \sqrt {2 + \sqrt 2 } } }} \times \frac{2}{{\sqrt {2 + \sqrt {2 + \sqrt {2 + \sqrt 2 } } } }} \times ...\]
\[\frac{\pi }{2} = \prod\limits_{n = 1}^\infty {\left( {\frac{{2n}}{{2n - 1}} \times \frac{{2n}}{{2n + 1}}} \right)} \]Giving
\[\frac{\pi }{2} = \frac{2}{1} \times \frac{2}{3} \times \frac{4}{3} \times \frac{4}{5} \times \frac{6}{5} \times \frac{6}{7} \times ...\]
\[\frac{\pi }{2} = \frac{2}{1} \times \frac{2}{3} \times \frac{4}{3} \times \frac{4}{5} \times \frac{6}{5} \times \frac{6}{7} \times ...\]
Newton's approximation. Sir Isaac Newton derived this formula in 1666, using it to calculate π to 16 places 'using 22 terms of the series' (in Pickover, 2008). In recognition of his obsessive calculations in the face of the irrationality of π, Newton wrote, 'I am ashamed to tell you to how many figures I carried these computations, having no other business at the time'. (Play with Newton's approximation using this interactive Wolfram Demonstration.)
\[\pi \approx \frac{3}{4}\sqrt 3 + 24\int\limits_0^{\frac{1}{4}} {\sqrt {x - {x^2}} } dx\]giving
\[\pi \approx \frac{3}{4}\sqrt 3 + 24\left( {\frac{1}{{12}} - \frac{1}{{5 \times {2^5}}} - \frac{1}{{28 \times {2^7}}} - \frac{1}{{72 \times {2^9}}} - ...} \right)\]
The Basel Problem. A famous problem (as outlined here by Marianne Freiberger in Plus magazine) named after the home town of the renowned Bernoullis who worked on it, and famously solved by the great Leonarhd Euler in 1735, giving the surprising π-related result (see this accessible outline by Chris Sangwin). Watch this superb video, from Grant Sanderson with Ben Hambrecht, giving a new take and beautiful proof of the problem, using light!
\[\frac{{{\pi ^2}}}{6} = \frac{1}{{{1^2}}} + \frac{1}{{{2^2}}} + \frac{1}{{{3^2}}} + \frac{1}{{{4^2}}} + ...\]
\[\pi \approx \frac{3}{4}\sqrt 3 + 24\left( {\frac{1}{{12}} - \frac{1}{{5 \times {2^5}}} - \frac{1}{{28 \times {2^7}}} - \frac{1}{{72 \times {2^9}}} - ...} \right)\]
Machin's formula. Published in William Jones' 1706 book Synopsis palmariorum matheseos, the same book where π was first used to denote the ratio of a circle's circumference to its diameter, Machin's rapidly converging series allowed π to be computed to 100 decimal places. (To see how Machin's formula converges to π, play with this interactive Wolfram Demonstration.)
\[\frac{\pi }{4} = 4{\tan ^{ - 1}}\left( {\frac{1}{5}} \right) - {\tan ^{ - 1}}\left( {\frac{1}{{239}}} \right)\]Or,
\[\frac{\pi }{4} = 4{\cot ^{ - 1}}\left( 5 \right) - {\cot ^{ - 1}}\left( {239} \right)\]
\[\frac{\pi }{4} = 4{\tan ^{ - 1}}\left( {\frac{1}{5}} \right) - {\tan ^{ - 1}}\left( {\frac{1}{{239}}} \right)\]Or,
\[\frac{\pi }{4} = 4{\cot ^{ - 1}}\left( 5 \right) - {\cot ^{ - 1}}\left( {239} \right)\]
\[\frac{{{\pi ^2}}}{6} = \frac{1}{{{1^2}}} + \frac{1}{{{2^2}}} + \frac{1}{{{3^2}}} + \frac{1}{{{4^2}}} + ...\]
Ramanuja's formula. Ramanujan's remarkable infinite series for π (from his first published paper of 1914) converges incredibly fast:
\[\frac{1}{\pi } = \frac{{2\sqrt 2 }}{{9801}}\sum\limits_{k = 0}^\infty {\frac{{\left( {4k} \right)!\left( {1103 + 26390k} \right)}}{{{{\left( {k!} \right)}^4}{{396}^{4k}}}}} \]
When k = 0, for example, the formula becomes:
\[\pi \approx \frac{{9801}}{{2206\sqrt 2 }} = 3.14159273001...\]
which is π correct to 6 decimal places (error = 0.0000000764235...). Each successive term of the formula adds around another 8 decimal places to the correct value of π. The second iteration produces a value for correct to 15 decimal places:
\[\pi \approx \frac{{2510613731736\sqrt 2 }}{{27493}} + \frac{{9801}}{{2206\sqrt 2 }} + = 3.1415926535897938779...\]
42 Billion digits. This formula, from Lennart Berggren and Jonathan and Peter Borwein in 1997 (see the 2004 3rd edition of their book in the references) yields π correct to an astonishing 42 billion digits. Professor Thomas Osler considered the formula in more detail in this paper.
\[{\left( {\frac{1}{{{{10}^5}}}\sum\limits_{n = - \infty }^{n = \infty } {{e^{\frac{{ - {n^2}}}{{{{10}^{10}}}}}}} } \right)^2} \approx \pi \]
On π-day 2024 (or rather, at some point on π-day 2024):
- A 3 year old born on 22 January 2021 will be π.
- A 6 year old born on 1 December 2017 will be 2π.
- A 9 year old born on 10 October 2014 will be 3π.
- A 9 year old born on 1 May 2014 will be \({\pi ^2}\) (or ππ).
- A 12 year old born on 20 August 2011 will be 4π.
- A 15 year old born on 29 June 2008 will be 5π.
- A 36 year old born on 28 September 1987 will be \({\pi ^\pi }\).
If you teach in a primary, make sure to find students in your school born on 1 May 2014 (Y5), 10 October 2014 (Y4), and 1 December 2017 (Y2), and make a fuss of their ππ, 3π and 2π birthdays respectively! They may not know what π is yet, but this is a lovely way to sew a seed of interest and give them something to take home and surprise their parents with. If you teach in a secondary, make sure to find students in your school born on 20 August 2011 (Y8) and 29 June 2008 (Y11) and make a fuss of their 4π and 5π birthdays respectively. And of course, if you have any 36 year old colleagues, friends or family born on 28 September 1987, make sure you make a fuss of their \({\pi ^\pi }\) birthdays!
You may of course be celebrating a π-related birthday on a day different to π-day. A complete list of π, 2π, 3π, ππ, 4π, 5π and \({\pi ^\pi }\) birthdays for each day of 2024, not just for π-day, can be viewed here. If you know anyone lucky enough to be marking such a birthday this year, make sure you put it in your diary and surprise them on the day with a HapPi Birthday wish.
Famous π-day birthdays
(Click here to return to the menu)
The fact that Albert Einstein was born on π-day 1879 in Ulm, Germany, is well-known, and rightly celebrated. Less known, however, and less celebrated, is the fact that the renowned Polish mathematician, Wacław Sierpiński, was born on π-day just \(\left\lceil \pi \right\rceil \pi \) years later in 1882 in Warszawa, Poland.
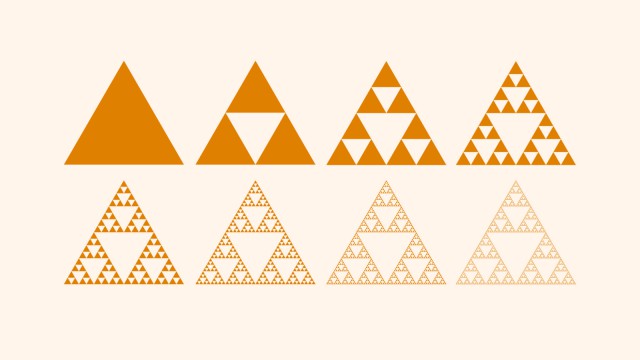
Sierpiński made groundbreaking contributions to set theory, number theory, functions and topology, and has a number of well-known fractal objects named after him that are interesting to explore with students when exploring iterative procedures and nth term generalisations, as well as, of course, when playing around with the idea of infinity. Three of these Sierpiński fractal objects are the Sierpiński carpet, the Sierpiński triangle (also referred to as the Sierpiński sieve or gasket), and the Sierpiński curve (including the Sierpiński arrowhead curve). The first seven iterations of the Sierpiński carpet and Sierpiński triangle are shown in the images below. An interesting problem to consider with students is to find the area of the carpet and triangle after 1, 2, 3, ...n iterations.
Taking the carpet above as an example, assuming the original square has a side length of 1 and thus an area of 1, after the 1st iteration the shaded area is \({\raise0.5ex\hbox{$\scriptstyle 8$}
\kern-0.1em/\kern-0.15em
\lower0.25ex\hbox{$\scriptstyle 9$}}\) of 1. Visualising this 1st iteration as being made up of 8 smaller shaded squares, i.e. each with a side length of \({\raise0.5ex\hbox{$\scriptstyle 1$}
\kern-0.1em/\kern-0.15em
\lower0.25ex\hbox{$\scriptstyle 3$}}\), we can see that the area of each of these smaller squares after the subsequent 2nd iteration is \({\raise0.5ex\hbox{$\scriptstyle 8$}
\kern-0.1em/\kern-0.15em
\lower0.25ex\hbox{$\scriptstyle 9$}}\) of \({\raise0.5ex\hbox{$\scriptstyle 1$}
\kern-0.1em/\kern-0.15em
\lower0.25ex\hbox{$\scriptstyle 9$}}\), but there are 8 of these squares, thus meaning that after the second iteration the area of the original starting square that is shaded is \({\raise0.5ex\hbox{$\scriptstyle 8$}
\kern-0.1em/\kern-0.15em
\lower0.25ex\hbox{$\scriptstyle 9$}} \times {\raise0.5ex\hbox{$\scriptstyle 8$}
\kern-0.1em/\kern-0.15em
\lower0.25ex\hbox{$\scriptstyle 9$}} = {\left( {{\raise0.5ex\hbox{$\scriptstyle 8$}
\kern-0.1em/\kern-0.15em
\lower0.25ex\hbox{$\scriptstyle 9$}}} \right)^2}\). And so on, such that after n iterations, the area of the original square \({A_n}\) is
\[{A_n} = {\left( {\frac{8}{9}} \right)^n}\]
Students could ponder what the area of the carpet is exactly, when \(n = \infty \), i.e. after an infinite number of iterations (this problem was solved in 2012 by Yaroslav Sergeyev, as revealed in this paper).
The greatest π-day birthday in history
(Click here to return to the menu)
In 1592, it is possible (probable perhaps) that someone was π years old, at π o'clock precisely, on what we now know as π-day. Or to put it another way, it is likely that at least one person was 3.141592653... years old on 3/14/1592 (mm/dd form) at 3:14am and 15.92653... seconds (or at 3.141592653... am). So, when was this person born? (Click here for the problem download, a solution think through, and the name of someone who would have been π years old at some point on π-day in 1592!)
π o'clock
(Click here to return to the menu)
You might like to try out this problem from my blog with students. The clock face implies for students what is meant by π o’clock in the problem, but perhaps it could mean something else, e.g. 3.141592... may suggest 3 hours and 0.141519... of an hour, rather than the 3:14 and 15.92 seconds the clock implies. How accurate could students calculate the angle, i.e. will they use π to 4, 6, 8, 10... decimal places?
(Click here to return to the menu)
The fact that Albert Einstein was born on π-day 1879 in Ulm, Germany, is well-known, and rightly celebrated. Less known, however, and less celebrated, is the fact that the renowned Polish mathematician, Wacław Sierpiński, was born on π-day just \(\left\lceil \pi \right\rceil \pi \) years later in 1882 in Warszawa, Poland.
Sierpiński made groundbreaking contributions to set theory, number theory, functions and topology, and has a number of well-known fractal objects named after him that are interesting to explore with students when exploring iterative procedures and nth term generalisations, as well as, of course, when playing around with the idea of infinity. Three of these Sierpiński fractal objects are the Sierpiński carpet, the Sierpiński triangle (also referred to as the Sierpiński sieve or gasket), and the Sierpiński curve (including the Sierpiński arrowhead curve). The first seven iterations of the Sierpiński carpet and Sierpiński triangle are shown in the images below. An interesting problem to consider with students is to find the area of the carpet and triangle after 1, 2, 3, ...n iterations.
Taking the carpet above as an example, assuming the original square has a side length of 1 and thus an area of 1, after the 1st iteration the shaded area is \({\raise0.5ex\hbox{$\scriptstyle 8$}
\kern-0.1em/\kern-0.15em
\lower0.25ex\hbox{$\scriptstyle 9$}}\) of 1. Visualising this 1st iteration as being made up of 8 smaller shaded squares, i.e. each with a side length of \({\raise0.5ex\hbox{$\scriptstyle 1$}
\kern-0.1em/\kern-0.15em
\lower0.25ex\hbox{$\scriptstyle 3$}}\), we can see that the area of each of these smaller squares after the subsequent 2nd iteration is \({\raise0.5ex\hbox{$\scriptstyle 8$}
\kern-0.1em/\kern-0.15em
\lower0.25ex\hbox{$\scriptstyle 9$}}\) of \({\raise0.5ex\hbox{$\scriptstyle 1$}
\kern-0.1em/\kern-0.15em
\lower0.25ex\hbox{$\scriptstyle 9$}}\), but there are 8 of these squares, thus meaning that after the second iteration the area of the original starting square that is shaded is \({\raise0.5ex\hbox{$\scriptstyle 8$}
\kern-0.1em/\kern-0.15em
\lower0.25ex\hbox{$\scriptstyle 9$}} \times {\raise0.5ex\hbox{$\scriptstyle 8$}
\kern-0.1em/\kern-0.15em
\lower0.25ex\hbox{$\scriptstyle 9$}} = {\left( {{\raise0.5ex\hbox{$\scriptstyle 8$}
\kern-0.1em/\kern-0.15em
\lower0.25ex\hbox{$\scriptstyle 9$}}} \right)^2}\). And so on, such that after n iterations, the area of the original square \({A_n}\) is
\[{A_n} = {\left( {\frac{8}{9}} \right)^n}\]
Students could ponder what the area of the carpet is exactly, when \(n = \infty \), i.e. after an infinite number of iterations (this problem was solved in 2012 by Yaroslav Sergeyev, as revealed in this paper).
(Click here to return to the menu)
In 1592, it is possible (probable perhaps) that someone was π years old, at π o'clock precisely, on what we now know as π-day. Or to put it another way, it is likely that at least one person was 3.141592653... years old on 3/14/1592 (mm/dd form) at 3:14am and 15.92653... seconds (or at 3.141592653... am). So, when was this person born? (Click here for the problem download, a solution think through, and the name of someone who would have been π years old at some point on π-day in 1592!)
(Click here to return to the menu)
You might like to try out this problem from my blog with students. The clock face implies for students what is meant by π o’clock in the problem, but perhaps it could mean something else, e.g. 3.141592... may suggest 3 hours and 0.141519... of an hour, rather than the 3:14 and 15.92 seconds the clock implies. How accurate could students calculate the angle, i.e. will they use π to 4, 6, 8, 10... decimal places?
π is the 73rd day of the year
(Click here to return to the menu)
73 is a permutable (or anagrammatic) prime, a prime that can have its digits' rearranged (in base 10) in any permutation and still be a prime number. 73 is also an emirp, a number whose reverse, 37, is also prime — a property also evident in terms of the respective ordinal positions of 73 and its emirp partner, 73 being the 21st prime while 37 is the 12th prime. 73 is a Sophie Germain prime and is palindromic in binary 1001001 (interestingly, all Fermat primes and Mersenne primes are subsets of the binary palindromic primes). 73 is also an octal palindrome 111 and the only octal prime repunit. 73 is, moreover, and for some of the reasons given here, Sheldon Cooper's favourite integer in The Big Bang Theory — as was first referenced in the show’s 73rd episode. (Jim Parsons, incidentally, the actor who plays Sheldon Cooper, was born in 1973.) 73 is also the number that marks when English-speaking children have learned the rules of counting sufficiently to overcome the cognitive need for memorisation, thus implying that once you can count to 73 in English, you can count forever (see this post for more detail about 73).
π primes
(Click here to return to the menu)
A pi-prime (sequence A005042 in the OEIS) is a prime number made up of the initial digits of the decimal expansion of π. To date we have found the first four pi-primes (sequence A060421), with another four found to be probable (the largest having 613,373 digits). The first four pi-primes are:
\[\begin{array}{l}3,\\31,\\314159,\\31415926535897932384626433832795028841\end{array}\]
The fifth, a probable prime, 16,208 digits long, took four and a half months to compute:
31415926535897932384626433832795028841971693993751058209749445923078164062862089986280348253421170679821480865132823066470938446095505822317253594081284811174502841027019385211055596446229489549303819644288109756659334461284756482337867831652712019091456485669234603486104543266482133936072602491412737245870066063155881748815209209628292540917153643678925903600113305305488204665213841469519415116094330572703657595919530921861173819326117931051185480744623799627495673518857527248912279381830119491298336733624406566430860213949463952247371907021798609437027705392171762931767523846748184676694051320005681271452635608277857713427577896091736371787214684409012249534301465495853710507922796892589235420199561121290219608640344181598136297747713099605187072113499999983729780499510597317328160963185950244594553469083026425223082533446850352619311881710100031378387528865875332083814206171776691473035982534904287554687311595628638823537875937519577818577805321712268066130019278766111959092164201989380952572010654858632788659361533818279682303019520353018529689957736225994138912497217752834791315155748572424541506959508295331168617278558890750983817546374649393192550604009277016711390098488240128583616035637076601047101819429555961989467678374494482553797747268471040475346462080466842590694912933136770289891521047521620569660240580381501935112533824300355876402474964732639141992726042699227967823547816360093417216412199245863150302861829745557067498385054945885869269956909272107975093029553211653449872027559602364806654991198818347977535663698074265425278625518184175746728909777727938000816470600161452491921732172147723501414419735685481613611573525521334757418494684385233239073941433345477624168625189835694855620992192221842725502542568876717904946016534668049886272327917860857843838279679766814541009538837863609506800642251252051173929848960841284886269456042419652850222106611863067442786220391949450471237137869609563643719172874677646575739624138908658326459958133904780275900994657640789512694683983525957098258226205224894077267194782684826014769909026401363944374553050682034962524517493996514314298091906592509372216964615157098583874105978859597729754989301617539284681382686838689427741559918559252459539594310499725246808459872736446958486538367362226260991246080512438843904512441365497627807977156914359977001296160894416948685558484063534220722258284886481584560285060168427394522674676788952521385225499546667278239864565961163548862305774564980355936345681743241125150760694794510965960940252288797108931456691368672287489405601015033086179286809208747609178249385890097149096759852613655497818931297848216829989487226588048575640142704775551323796414515237462343645428584447952658678210511413547357395231134271661021359695362314429524849371871101457654035902799344037420073105785390621983874478084784896833214457138687519435064302184531910484810053706146806749192781911979399520614196634287544406437451237181921799983910159195618146751426912397489409071864942319615679452080951465502252316038819301420937621378559566389377870830390697920773467221825625996615014215030680384477345492026054146659252014974428507325186660021324340881907104863317346496514539057962685610055081066587969981635747363840525714591028970641401109712062804390397595156771577004203378699360072305587631763594218731251471205329281918261861258673215791984148488291644706095752706957220917567116722910981690915280173506712748583222871835209353965725121083579151369882091444210067510334671103141267111369908658516398315019701651511685171437657618351556508849099898599823873455283316355076479185358932261854896321329330898570642046752590709154814165498594616371802709819943099244889575712828905923233260972997120844335732654893823911932597463667305836041428138830320382490375898524374417029132765618093773444030707469211201913020330380197621101100449293215160842444859637669838952286847831235526582131449576857262433441893039686426243410773226978028073189154411010446823252716201052652272111660396665573092547110557853763466820653109896526918620564769312570586356620185581007293606598764861179104533488503461136576867532494416680396265797877185560845529654126654085306143444318586769751456614068007002378776591344017127494704205622305389945613140711270004078547332699390814546646458807972708266830634328587856983052358089330657574067954571637752542021149557615814002501262285941302164715509792592309907965473761255176567513575178296664547791745011299614890304639947132962107340437518957359614589019389713111790429782856475032031986915140287080859904801094121472213179476477726224142548545403321571853061422881375850430633217518297986622371721591607716692547487389866549494501146540628433663937900397692656721463853067360965712091807638327166416274888800786925602902284721040317211860820419000422966171196377921337575114959501566049631862947265473642523081770367515906735023507283540567040386743513622224771589150495309844489333096340878076932599397805419341447377441842631298608099888687413260472156951623965864573021631598193195167353812974167729478672422924654366800980676928238280689964004824354037014163149658979409243237896907069779422362508221688957383798623001593776471651228935786015881617557829735233446042815126272037343146531977774160319906655418763979293344195215413418994854447345673831624993419131814809277771038638773431772075456545322077709212019051660962804909263601975988281613323166636528619326686336062735676303544776280350450777235547105859548702790814356240145171806246436267945612753181340783303362542327839449753824372058353114771199260638133467768796959703098339130771098704085913374641442822772634659470474587847787201927715280731767907707157213444730605700733492436931138350493163128404251219256517980694113528013147013047816437885185290928545201165839341965621349143415956258658655705526904965209858033850722426482939728584783163057777560688876446248246857926039535277348030480290058760758251047470916439613626760449256274204208320856611906254543372131535958450687724602901618766795240616342522577195429162991930645537799140373404328752628889639958794757291746426357455254079091451357111369410911939325191076020825202618798531887705842972591677813149699009019211697173727847684726860849003377024242916513005005168323364350389517029893922334517220138128069650117844087451960121228599371623130171144484640903890644954440061986907548516026327505298349187407866808818338510228334508504860825039302133219715518430635455007668282949304137765527939751754613953984683393638304746119966538581538420568533862186725233402830871123282789212507712629463229563989898935821167456270102183564622013496715188190973038119800497340723961036854066431939509790190699639552453005450580685501956730229219139339185680344903982059551002263535361920419947455385938102343955449597783779023742161727111723643435439478221818528624085140066604433258885698670543154706965747458550332323342107301545940516553790686627333799585115625784322988273723198987571415957811196358330059408730681216028764962867446047746491599505497374256269010490377819868359381465741268049256487985561453723478673303904688383436346553794986419270563872931748723320837601123029911367938627089438799362016295154133714248928307220126901475466847653576164773794675200490757155527819653621323926406160136358155907422020203187277605277219005561484255518792530343513984425322341576233610642506390497500865627109535919465897514131034822769306247435363256916078154781811528436679570611086153315044521274739245449454236828860613408414863776700961207151249140430272538607648236341433462351897576645216413767969031495019108575984423919862916421939949072362346468441173940326591840443780513338945257423995082965912285085558215725031071257012668302402929525220118726767562204154205161841634847565169998116141010029960783869092916030288400269104140792886215078424516709087000699282120660418371806535567252532567532861291042487761825829765157959847035622262934860034158722980534989650226291748788202734209222245339856264766914905562842503912757710284027998066365825488926488025456610172967026640765590429099456815065265305371829412703369313785178609040708667114965583434347693385781711386455873678123014587687126603489139095620099393610310291616152881384379099042317473363948045759314931405297634757481193567091101377517210080315590248530906692037671922033229094334676851422144773793937517034436619910403375111735471918550464490263655128162288244625759163330391072253837421821408835086573917715096828874782656995995744906617583441375223970968340800535598491754173818839994469748676265516582765848358845314277568790029095170283529716344562129640435231176006651012412006597558512761785838292041974844236080071930457618932349229279650198751872127267507981255470958904556357921221033346697499235630254947802490114195212382815309114079073860251522742995818072471625916685451333123948049470791191532673430282441860414263639548000448002670496248201792896476697583183271314251702969234889627668440323260927524960357996469256504936818360900323809293459588970695365349406034021665443755890045632882250545255640564482465151875471196218443965825337543885690941130315095261793780029741207665147939425902989695946995565761218656196733786236256125216320862869222103274889218654364802296780705765615144632046927906821207388377814233562823608963208068222468012248261177185896381409183903673672220888321513755600372798394004152970028783076670944474560134556417254370906979396122571429894671543578468788614445812314593571984922528471605049221242470141214780573455105008019086996033027634787081081754501193071412233908663938339529425786905076431006383519834389341596131854347546495569781038293097164651438407007073604112373599843452251610507027056235266012764848308407611830130527932054274628654036036745328651057065874882256981579367897669742205750596834408697350201410206723585020072452256326513410559240190274216248439140359989535394590944070469120914093870012645600162374288021092764579310657922955249887275846101264836999892256959688159205600101655256375678566722796619885782794848855834397518744545512965634434803966420557982936804352202770984294232533022576341807039476994159791594530069752148293366555661567873640053666564165473217043903521329543529169414599041608753201868379370234888689479151071637852902345292440773659495630510074210871426134974595615138498713757047101787957310422969066670214498637464595280824369445789772330048764765241339075920434019634039114732023380715095222010682563427471646024335440051521266932493419673977041595683753555166730273900749729736354964533288869844061196496162773449518273695588220757355176651589855190986665393549481068873206859907540792342402300925900701731960362254756478940647548346647760411463233905651343306844953979070903023460461470961696886885014083470405460742958699138296682468185710318879065287036650832431974404771855678934823089431068287027228097362480939962706074726455399253994428081137369433887294063079261595995462624629707062594845569034711972996409089418059534393251236235508134949004364278527138315912568989295196427287573946914272534366941532361004537304881985517065941217352462589548730167600298865925786628561249665523533829428785425340483083307016537228563559152534784459818313411290019992059813522051173365856407826484942764411376393866924803118364453698589175442647399882284621844900877769776312795722672655562596282542765318300134070922334365779160128093179401718598599933849235495640057099558561134980252499066984233017350358044081168552653117099570899427328709258487894436460050410892266917835258707859512983441729535195378855345737426085902908176515578039059464087350612322611200937310804854852635722825768203416050484662775045003126200800799804925485346941469775164932709504934639382432227188515974054702148289711177792376122578873477188196825462981268685817050740272550263329044976277894423621674119186269439650671515779586756482399391760426017633870454990176143641204692182370764887834196896861181558158736062938603810171215855272668300823834046564758804051380801633638874216371406435495561868964112282140753302655100424104896783528588290243670904887118190909494533144218287661810310073547705498159680772009474696134360928614849417850171807793068108546900094458995279424398139213505586422196483491512639012803832001097738680662877923971801461343244572640097374257007359210031541508936793008169980536520276007277496745840028362405346037263416554259027601834840306811381855105979705664007509426087885735796037324514146786703688098806097164258497595138069309449401515422221943291302173912538355915031003330325111749156969174502714943315155885403922164097229101129035521815762823283182342548326111912800928252561902052630163911477247331485739107775874425387611746578671169414776421441111263583553871361011023267987756410246824032264834641766369806637857681349204530224081972785647198396308781543221166912246415911776732253264335686146186545222681268872684459684424161078540167681420808850280054143613146230821025941737562389942075713627516745731891894562835257044133543758575342698699472547031656613991999682628247270641336222178923903176085428943733935618891651250424404008952719837873864805847268954624388234375178852014395600571048119498842390606136957342315590796703461491434478863604103182350736502778590897578272731305048893989009923913503373250855982655867089242612429473670193907727130706869170926462548423240748550366080136046689511840093668609546325002145852930950000907151058236267293264537382104938724996699339424685516483261134146110680267446637334375340764294026682973865220935701626384648528514903629320199199688285171839536691345222444708045923966028171565515656661113598231122506289058549145097157553900243931535190902107119457300243880176615035270862602537881797519478061013715004489917210022201335013106016391541589578037117792775225978742891917915522417189585361680594741234193398420218745649256443462392531953135103311476394911995072858430658361935369329699289837914941939406085724863968836903265564364216644257607914710869984315733749648835292769328220762947282381537409961545598798259891093717126218283025848112389011968221429457667580718653806506487026133892822994972574530332838963818439447707794022843598834100358385423897354243956475556840952248445541392394100016207693636846776413017819659379971557468541946334893748439129742391433659360410035234377706588867781139498616478747140793263858738624732889645643598774667638479466504074111825658378878454858148962961273998413442726086061872455452360643153710112746809778704464094758280348769758948328241239292960582948619196670918958089833201210318430340128495116203534280144127617285830243559830032042024512072872535581195840149180969253395075778400067465526031446167050827682772223534191102634163157147406123850425845988419907611287258059113935689601431668283176323567325417073420817332230462987992804908514094790368878687894930546955703072619009502076433493359106024545086453628935456862958531315337183868265617862273637169757741830239860065914816164049449650117321313895747062088474802365371031150898427992754426853277974311395143574172219759799359685252285745263796289612691572357986620573408375766873884266405990993505000813375432454635967504844235284874701443545419576258473564216198134073468541117668831186544893776979566517279662326714810338643913751865946730024434500544995399742372328712494834706044063471606325830649829795510109541836235030309453097335834462839476304775645015008507578949548931393944899216125525597701436858943585877526379625597081677643800125436502371412783467926101995585224717220177723700417808419423948725406801556035998390548985723546745642390585850216719031395262944554391316631345308939062046784387785054239390524731362012947691874975191011472315289326772533918146607300089027768963114810902209724520759167297007850580717186381054967973100167870850694207092232908070383263453452038027860990556900134137182368370991949516489600755049341267876436746384902063964019766685592335654639138363185745698147196210841080961884605456039038455343729141446513474940784884423772175154334260306698831768331001133108690421939031080143784334151370924353013677631084913516156422698475074303297167469640666531527035325467112667522460551199581831963763707617991919203579582007595605302346267757943936307
Playing the π-day Lottery
(Click here to return to the menu)
This is (part of) the UK Lotto lottery ticket I bought for the 2018 π-day draw:
As you can see, the first set of numbers are the first twelve digits of π {31, 41, 59, 26, 53, 58}, and, moreover, the maximum number of digits from the start of π that can be used in the UK Lotto (six numbers from 1 to 59 are drawn). The second set of numbers are the numbers 1 to 6 {1, 2, 3, 4, 5, 6}. The third set of numbers are a randomly generated set {18, 58, 41, 11, 10, 31}. I didn't win.
Suggested explorations/diversions with/for students:
π as the Prime Counting Function
(Click here to return to the menu)
In 1909 Edmund Landau used the symbol π to describe Prime Counting Function \(\pi \left( x \right)\), which gives the number of primes not exceeding a given number \(x\). π used in this sense has nothing to do with π used in Jones' sense, i.e. π as Archimedes' constant, π as the ratio of a circle's circumference to its diameter. By way of illustration, \(\pi \left( 7 \right) = 4\) because the number of primes less than or equal to the number 7 is 4 (i.e. 2, 3, 5 and 7).
Suggested explorations/diversions with/for students:
Fake proofs for π
Memorising π
(Click here to return to the menu)
Daniel Tammet, essayist and novelist, is described as an autistic savant. Born with high-functioning autism, Daniel famously memorised and recited π to 22,514 places on π-day in 2004, taking him just over five hours to do so. This clip, lasting just under five minutes, is an excerpt from a longer documentary on Daniel, 'The Boy With The Incredible Brain'.
For more of Daniel:
Suresh Kumar Sharma of India recited 70,030 digits of π on 21 October 2015, taking 17 hours and 14 minutes to do so. See this article about the 'secret' to memorizing π to such huge amounts of digits. And see this article by @alexbellos in The Guardian about Akira Haraguch, who holds the unofficial World Record having recited π to 100,000 digits in October 2006, over 16 hours. It is fun to hold a class or school competition, linked to the 'self-referential stories' below perhaps, to see which student can recite π to the greatest amount of decimal places.
Pilish (Self-referential stories for π)
(Click here to return to the menu)
73 is a permutable (or anagrammatic) prime, a prime that can have its digits' rearranged (in base 10) in any permutation and still be a prime number. 73 is also an emirp, a number whose reverse, 37, is also prime — a property also evident in terms of the respective ordinal positions of 73 and its emirp partner, 73 being the 21st prime while 37 is the 12th prime. 73 is a Sophie Germain prime and is palindromic in binary 1001001 (interestingly, all Fermat primes and Mersenne primes are subsets of the binary palindromic primes). 73 is also an octal palindrome 111 and the only octal prime repunit. 73 is, moreover, and for some of the reasons given here, Sheldon Cooper's favourite integer in The Big Bang Theory — as was first referenced in the show’s 73rd episode. (Jim Parsons, incidentally, the actor who plays Sheldon Cooper, was born in 1973.) 73 is also the number that marks when English-speaking children have learned the rules of counting sufficiently to overcome the cognitive need for memorisation, thus implying that once you can count to 73 in English, you can count forever (see this post for more detail about 73).
(Click here to return to the menu)
A pi-prime (sequence A005042 in the OEIS) is a prime number made up of the initial digits of the decimal expansion of π. To date we have found the first four pi-primes (sequence A060421), with another four found to be probable (the largest having 613,373 digits). The first four pi-primes are:
\[\begin{array}{l}3,\\31,\\314159,\\31415926535897932384626433832795028841\end{array}\]
The fifth, a probable prime, 16,208 digits long, took four and a half months to compute:
31415926535897932384626433832795028841971693993751058209749445923078164062862089986280348253421170679821480865132823066470938446095505822317253594081284811174502841027019385211055596446229489549303819644288109756659334461284756482337867831652712019091456485669234603486104543266482133936072602491412737245870066063155881748815209209628292540917153643678925903600113305305488204665213841469519415116094330572703657595919530921861173819326117931051185480744623799627495673518857527248912279381830119491298336733624406566430860213949463952247371907021798609437027705392171762931767523846748184676694051320005681271452635608277857713427577896091736371787214684409012249534301465495853710507922796892589235420199561121290219608640344181598136297747713099605187072113499999983729780499510597317328160963185950244594553469083026425223082533446850352619311881710100031378387528865875332083814206171776691473035982534904287554687311595628638823537875937519577818577805321712268066130019278766111959092164201989380952572010654858632788659361533818279682303019520353018529689957736225994138912497217752834791315155748572424541506959508295331168617278558890750983817546374649393192550604009277016711390098488240128583616035637076601047101819429555961989467678374494482553797747268471040475346462080466842590694912933136770289891521047521620569660240580381501935112533824300355876402474964732639141992726042699227967823547816360093417216412199245863150302861829745557067498385054945885869269956909272107975093029553211653449872027559602364806654991198818347977535663698074265425278625518184175746728909777727938000816470600161452491921732172147723501414419735685481613611573525521334757418494684385233239073941433345477624168625189835694855620992192221842725502542568876717904946016534668049886272327917860857843838279679766814541009538837863609506800642251252051173929848960841284886269456042419652850222106611863067442786220391949450471237137869609563643719172874677646575739624138908658326459958133904780275900994657640789512694683983525957098258226205224894077267194782684826014769909026401363944374553050682034962524517493996514314298091906592509372216964615157098583874105978859597729754989301617539284681382686838689427741559918559252459539594310499725246808459872736446958486538367362226260991246080512438843904512441365497627807977156914359977001296160894416948685558484063534220722258284886481584560285060168427394522674676788952521385225499546667278239864565961163548862305774564980355936345681743241125150760694794510965960940252288797108931456691368672287489405601015033086179286809208747609178249385890097149096759852613655497818931297848216829989487226588048575640142704775551323796414515237462343645428584447952658678210511413547357395231134271661021359695362314429524849371871101457654035902799344037420073105785390621983874478084784896833214457138687519435064302184531910484810053706146806749192781911979399520614196634287544406437451237181921799983910159195618146751426912397489409071864942319615679452080951465502252316038819301420937621378559566389377870830390697920773467221825625996615014215030680384477345492026054146659252014974428507325186660021324340881907104863317346496514539057962685610055081066587969981635747363840525714591028970641401109712062804390397595156771577004203378699360072305587631763594218731251471205329281918261861258673215791984148488291644706095752706957220917567116722910981690915280173506712748583222871835209353965725121083579151369882091444210067510334671103141267111369908658516398315019701651511685171437657618351556508849099898599823873455283316355076479185358932261854896321329330898570642046752590709154814165498594616371802709819943099244889575712828905923233260972997120844335732654893823911932597463667305836041428138830320382490375898524374417029132765618093773444030707469211201913020330380197621101100449293215160842444859637669838952286847831235526582131449576857262433441893039686426243410773226978028073189154411010446823252716201052652272111660396665573092547110557853763466820653109896526918620564769312570586356620185581007293606598764861179104533488503461136576867532494416680396265797877185560845529654126654085306143444318586769751456614068007002378776591344017127494704205622305389945613140711270004078547332699390814546646458807972708266830634328587856983052358089330657574067954571637752542021149557615814002501262285941302164715509792592309907965473761255176567513575178296664547791745011299614890304639947132962107340437518957359614589019389713111790429782856475032031986915140287080859904801094121472213179476477726224142548545403321571853061422881375850430633217518297986622371721591607716692547487389866549494501146540628433663937900397692656721463853067360965712091807638327166416274888800786925602902284721040317211860820419000422966171196377921337575114959501566049631862947265473642523081770367515906735023507283540567040386743513622224771589150495309844489333096340878076932599397805419341447377441842631298608099888687413260472156951623965864573021631598193195167353812974167729478672422924654366800980676928238280689964004824354037014163149658979409243237896907069779422362508221688957383798623001593776471651228935786015881617557829735233446042815126272037343146531977774160319906655418763979293344195215413418994854447345673831624993419131814809277771038638773431772075456545322077709212019051660962804909263601975988281613323166636528619326686336062735676303544776280350450777235547105859548702790814356240145171806246436267945612753181340783303362542327839449753824372058353114771199260638133467768796959703098339130771098704085913374641442822772634659470474587847787201927715280731767907707157213444730605700733492436931138350493163128404251219256517980694113528013147013047816437885185290928545201165839341965621349143415956258658655705526904965209858033850722426482939728584783163057777560688876446248246857926039535277348030480290058760758251047470916439613626760449256274204208320856611906254543372131535958450687724602901618766795240616342522577195429162991930645537799140373404328752628889639958794757291746426357455254079091451357111369410911939325191076020825202618798531887705842972591677813149699009019211697173727847684726860849003377024242916513005005168323364350389517029893922334517220138128069650117844087451960121228599371623130171144484640903890644954440061986907548516026327505298349187407866808818338510228334508504860825039302133219715518430635455007668282949304137765527939751754613953984683393638304746119966538581538420568533862186725233402830871123282789212507712629463229563989898935821167456270102183564622013496715188190973038119800497340723961036854066431939509790190699639552453005450580685501956730229219139339185680344903982059551002263535361920419947455385938102343955449597783779023742161727111723643435439478221818528624085140066604433258885698670543154706965747458550332323342107301545940516553790686627333799585115625784322988273723198987571415957811196358330059408730681216028764962867446047746491599505497374256269010490377819868359381465741268049256487985561453723478673303904688383436346553794986419270563872931748723320837601123029911367938627089438799362016295154133714248928307220126901475466847653576164773794675200490757155527819653621323926406160136358155907422020203187277605277219005561484255518792530343513984425322341576233610642506390497500865627109535919465897514131034822769306247435363256916078154781811528436679570611086153315044521274739245449454236828860613408414863776700961207151249140430272538607648236341433462351897576645216413767969031495019108575984423919862916421939949072362346468441173940326591840443780513338945257423995082965912285085558215725031071257012668302402929525220118726767562204154205161841634847565169998116141010029960783869092916030288400269104140792886215078424516709087000699282120660418371806535567252532567532861291042487761825829765157959847035622262934860034158722980534989650226291748788202734209222245339856264766914905562842503912757710284027998066365825488926488025456610172967026640765590429099456815065265305371829412703369313785178609040708667114965583434347693385781711386455873678123014587687126603489139095620099393610310291616152881384379099042317473363948045759314931405297634757481193567091101377517210080315590248530906692037671922033229094334676851422144773793937517034436619910403375111735471918550464490263655128162288244625759163330391072253837421821408835086573917715096828874782656995995744906617583441375223970968340800535598491754173818839994469748676265516582765848358845314277568790029095170283529716344562129640435231176006651012412006597558512761785838292041974844236080071930457618932349229279650198751872127267507981255470958904556357921221033346697499235630254947802490114195212382815309114079073860251522742995818072471625916685451333123948049470791191532673430282441860414263639548000448002670496248201792896476697583183271314251702969234889627668440323260927524960357996469256504936818360900323809293459588970695365349406034021665443755890045632882250545255640564482465151875471196218443965825337543885690941130315095261793780029741207665147939425902989695946995565761218656196733786236256125216320862869222103274889218654364802296780705765615144632046927906821207388377814233562823608963208068222468012248261177185896381409183903673672220888321513755600372798394004152970028783076670944474560134556417254370906979396122571429894671543578468788614445812314593571984922528471605049221242470141214780573455105008019086996033027634787081081754501193071412233908663938339529425786905076431006383519834389341596131854347546495569781038293097164651438407007073604112373599843452251610507027056235266012764848308407611830130527932054274628654036036745328651057065874882256981579367897669742205750596834408697350201410206723585020072452256326513410559240190274216248439140359989535394590944070469120914093870012645600162374288021092764579310657922955249887275846101264836999892256959688159205600101655256375678566722796619885782794848855834397518744545512965634434803966420557982936804352202770984294232533022576341807039476994159791594530069752148293366555661567873640053666564165473217043903521329543529169414599041608753201868379370234888689479151071637852902345292440773659495630510074210871426134974595615138498713757047101787957310422969066670214498637464595280824369445789772330048764765241339075920434019634039114732023380715095222010682563427471646024335440051521266932493419673977041595683753555166730273900749729736354964533288869844061196496162773449518273695588220757355176651589855190986665393549481068873206859907540792342402300925900701731960362254756478940647548346647760411463233905651343306844953979070903023460461470961696886885014083470405460742958699138296682468185710318879065287036650832431974404771855678934823089431068287027228097362480939962706074726455399253994428081137369433887294063079261595995462624629707062594845569034711972996409089418059534393251236235508134949004364278527138315912568989295196427287573946914272534366941532361004537304881985517065941217352462589548730167600298865925786628561249665523533829428785425340483083307016537228563559152534784459818313411290019992059813522051173365856407826484942764411376393866924803118364453698589175442647399882284621844900877769776312795722672655562596282542765318300134070922334365779160128093179401718598599933849235495640057099558561134980252499066984233017350358044081168552653117099570899427328709258487894436460050410892266917835258707859512983441729535195378855345737426085902908176515578039059464087350612322611200937310804854852635722825768203416050484662775045003126200800799804925485346941469775164932709504934639382432227188515974054702148289711177792376122578873477188196825462981268685817050740272550263329044976277894423621674119186269439650671515779586756482399391760426017633870454990176143641204692182370764887834196896861181558158736062938603810171215855272668300823834046564758804051380801633638874216371406435495561868964112282140753302655100424104896783528588290243670904887118190909494533144218287661810310073547705498159680772009474696134360928614849417850171807793068108546900094458995279424398139213505586422196483491512639012803832001097738680662877923971801461343244572640097374257007359210031541508936793008169980536520276007277496745840028362405346037263416554259027601834840306811381855105979705664007509426087885735796037324514146786703688098806097164258497595138069309449401515422221943291302173912538355915031003330325111749156969174502714943315155885403922164097229101129035521815762823283182342548326111912800928252561902052630163911477247331485739107775874425387611746578671169414776421441111263583553871361011023267987756410246824032264834641766369806637857681349204530224081972785647198396308781543221166912246415911776732253264335686146186545222681268872684459684424161078540167681420808850280054143613146230821025941737562389942075713627516745731891894562835257044133543758575342698699472547031656613991999682628247270641336222178923903176085428943733935618891651250424404008952719837873864805847268954624388234375178852014395600571048119498842390606136957342315590796703461491434478863604103182350736502778590897578272731305048893989009923913503373250855982655867089242612429473670193907727130706869170926462548423240748550366080136046689511840093668609546325002145852930950000907151058236267293264537382104938724996699339424685516483261134146110680267446637334375340764294026682973865220935701626384648528514903629320199199688285171839536691345222444708045923966028171565515656661113598231122506289058549145097157553900243931535190902107119457300243880176615035270862602537881797519478061013715004489917210022201335013106016391541589578037117792775225978742891917915522417189585361680594741234193398420218745649256443462392531953135103311476394911995072858430658361935369329699289837914941939406085724863968836903265564364216644257607914710869984315733749648835292769328220762947282381537409961545598798259891093717126218283025848112389011968221429457667580718653806506487026133892822994972574530332838963818439447707794022843598834100358385423897354243956475556840952248445541392394100016207693636846776413017819659379971557468541946334893748439129742391433659360410035234377706588867781139498616478747140793263858738624732889645643598774667638479466504074111825658378878454858148962961273998413442726086061872455452360643153710112746809778704464094758280348769758948328241239292960582948619196670918958089833201210318430340128495116203534280144127617285830243559830032042024512072872535581195840149180969253395075778400067465526031446167050827682772223534191102634163157147406123850425845988419907611287258059113935689601431668283176323567325417073420817332230462987992804908514094790368878687894930546955703072619009502076433493359106024545086453628935456862958531315337183868265617862273637169757741830239860065914816164049449650117321313895747062088474802365371031150898427992754426853277974311395143574172219759799359685252285745263796289612691572357986620573408375766873884266405990993505000813375432454635967504844235284874701443545419576258473564216198134073468541117668831186544893776979566517279662326714810338643913751865946730024434500544995399742372328712494834706044063471606325830649829795510109541836235030309453097335834462839476304775645015008507578949548931393944899216125525597701436858943585877526379625597081677643800125436502371412783467926101995585224717220177723700417808419423948725406801556035998390548985723546745642390585850216719031395262944554391316631345308939062046784387785054239390524731362012947691874975191011472315289326772533918146607300089027768963114810902209724520759167297007850580717186381054967973100167870850694207092232908070383263453452038027860990556900134137182368370991949516489600755049341267876436746384902063964019766685592335654639138363185745698147196210841080961884605456039038455343729141446513474940784884423772175154334260306698831768331001133108690421939031080143784334151370924353013677631084913516156422698475074303297167469640666531527035325467112667522460551199581831963763707617991919203579582007595605302346267757943936307
(Click here to return to the menu)
This is (part of) the UK Lotto lottery ticket I bought for the 2018 π-day draw:
As you can see, the first set of numbers are the first twelve digits of π {31, 41, 59, 26, 53, 58}, and, moreover, the maximum number of digits from the start of π that can be used in the UK Lotto (six numbers from 1 to 59 are drawn). The second set of numbers are the numbers 1 to 6 {1, 2, 3, 4, 5, 6}. The third set of numbers are a randomly generated set {18, 58, 41, 11, 10, 31}. I didn't win.
Suggested explorations/diversions with/for students:
- Find the probability of each separate set of numbers winning.
- Assuming one of the sets of numbers drawn in full, and thus wins the jackpot, which set would you expect to win the most money from?
- Whilst betting on the numbers 1, 2, 3, 4, 5 and 6 does not reduce your probability of winning, it does reduce the amount of money you are likely to win. This is because, perhaps surprisingly, the numbers 1, 2, 3, 4, 5 and 6 are selected by around 10,000 people each week, thus meaning that the winning jackpot would be shared by 10,000.
- Similarly, maybe a few mathsy people will buy a ticket with the first set of numbers on π-day, therefore reducing the amount of money you would win if drawn because of the shared jackpot.
- Find the combinations of all 'wins' you could possibly make with all three sets of numbers.
- You 'win' — relatively of course — in the Lotto if you match 6 numbers (Jackpot), or if you match 5 and the 'Bonus Ball', or if you match 5, 4, 3, or 2. (Visit the Lotto website for more detail.
- What is the least amount of initial digits from π that can be used in the UK Lotto?
- Find all the combinations of initial π digits that could buy a lottery ticket in the UK Lotto.
- Find the probability that a string of the initial digits of π will win the Lottery.
(Click here to return to the menu)
In 1909 Edmund Landau used the symbol π to describe Prime Counting Function \(\pi \left( x \right)\), which gives the number of primes not exceeding a given number \(x\). π used in this sense has nothing to do with π used in Jones' sense, i.e. π as Archimedes' constant, π as the ratio of a circle's circumference to its diameter. By way of illustration, \(\pi \left( 7 \right) = 4\) because the number of primes less than or equal to the number 7 is 4 (i.e. 2, 3, 5 and 7).
- What is the largest n you can find the value of \(\pi \left( n \right)\) for
- You may want to use the 'Sieve of Eratosthenes'
- The largest n for which a value of \(\pi \left( n \right)\) has been computed (by Staple 2015, as part of his Masters' degree) is \(\pi \left( {{{10}^{25}}} \right)\) = 1,699,246,750,872,437,141,327,603, which took 40,000 computing hours to find.
- Find the first five Ramanujan primes.
- A Ramanujan prime is the smallest number \({R_n}\) such that \(\pi \left( x \right) - \pi \left( {x/2} \right) \ge n\) for all \(x \ge {R_n}\).
(Click here to return to the menu)
These two now well-known fake 'proofs' are always interesting to share with students, in a 'surely this can't be right' sense. Firstly, this 'proof' that π = 4 always engages students visually, and is a nice conceit when thinking about gradients and derivatives (play with this Wolfram Demonstration for π = 4). Secondly, this following proof — which, on the face of it, seems fine to many — is a nice conceit when working with students on algebraic manipulation.
\[\begin{array}{c}\begin{align}x &= \frac{{\pi + 3}}{2}\\2x &= \pi + 3\\2x\left( {\pi - 3} \right) &= \left( {\pi + 3} \right)\left( {\pi - 3} \right)\\2\pi x - 6x &= {\pi ^2} - 3\pi + 3\pi - 9\\2\pi x - 6x &= {\pi ^2} - 9\\9 - 6x &= {\pi ^2} - 2\pi x\\9 - 6x + {x^2} &= {\pi ^2} - 2\pi x + {x^2}\\{\left( {3 - x} \right)^2} &= {\left( {\pi - x} \right)^2}\\3 - x &= \pi - x\\3 &= \pi \end{align}\end{array}\]
Suggested explorations/diversions with/for students:
These two now well-known fake 'proofs' are always interesting to share with students, in a 'surely this can't be right' sense. Firstly, this 'proof' that π = 4 always engages students visually, and is a nice conceit when thinking about gradients and derivatives (play with this Wolfram Demonstration for π = 4). Secondly, this following proof — which, on the face of it, seems fine to many — is a nice conceit when working with students on algebraic manipulation.
\[\begin{array}{c}\begin{align}x &= \frac{{\pi + 3}}{2}\\2x &= \pi + 3\\2x\left( {\pi - 3} \right) &= \left( {\pi + 3} \right)\left( {\pi - 3} \right)\\2\pi x - 6x &= {\pi ^2} - 3\pi + 3\pi - 9\\2\pi x - 6x &= {\pi ^2} - 9\\9 - 6x &= {\pi ^2} - 2\pi x\\9 - 6x + {x^2} &= {\pi ^2} - 2\pi x + {x^2}\\{\left( {3 - x} \right)^2} &= {\left( {\pi - x} \right)^2}\\3 - x &= \pi - x\\3 &= \pi \end{align}\end{array}\]
Suggested explorations/diversions with/for students:
- What is wrong with the 'proof' above?
- What happens when you 'fix' it?
- In this case, the problem comes from moving from \({\left( {3 - x} \right)^2} = {\left( {\pi - x} \right)^2}\) to \(3 - x = \pi - x\). The 'proof' only provides the positive square root, which produces the erroneous result.
- Make up you own fake π proof.
(Click here to return to the menu)
Daniel Tammet, essayist and novelist, is described as an autistic savant. Born with high-functioning autism, Daniel famously memorised and recited π to 22,514 places on π-day in 2004, taking him just over five hours to do so. This clip, lasting just under five minutes, is an excerpt from a longer documentary on Daniel, 'The Boy With The Incredible Brain'.
For more of Daniel:
- Watch How maths illuminates our lives, discussion with RSA (18m 23s)
- Watch Daniel On the language of numbers, on BBC Newsnight 2017 (5m 08s)
- Watch Daniel Learning to speak Icelandic in a week (6m 05s)
- Watch the documentary The Boy with the Incredible Brain in full (47m 24s)
- Read this lovely post from Maria Popova.
Milü — 密率 — the 4th convergent of the continued fraction for π, found by Tsu Ch'ung-Chihin in the 5th century AD, is the best rational approximation of π with a denominator of 4 digits or fewer, accurate to 6 decimal places, i.e., to within 0.000009%. For more on the beauty of continued fractions and π, the 'most romantic way to represent numbers', see this from Evelyn Lamb.
(Click here to return to the menu)
Arguably the best known π mnemonic, constructed by British astronomer Sir James Jeans, is: "How I want a drink, alcoholic of course, after the heavy chapters involving quantum mechanics", the number of letters in each word corresponding to the respective digit in the decimal expansion of π. Michael Keith proposed the name ‘Pilish’ for writing a sentence (or longer piece of poetry or prose) in which the lengths of successive words represent the digits of the number π, and his wonderful website is a treasure trove of Pilish literature, from plays, poems (called piems in Pilish), accidental Pilish and more. Keith's book, Not a Wake, is written entirely in Pilish from the first 10,000 digits π (the only book to have ever been written in Pilish: see this article from Alex Bellos for more). This, for example, from Keith in 1986, gives π to 356 places:
Arguably the best known π mnemonic, constructed by British astronomer Sir James Jeans, is: "How I want a drink, alcoholic of course, after the heavy chapters involving quantum mechanics", the number of letters in each word corresponding to the respective digit in the decimal expansion of π. Michael Keith proposed the name ‘Pilish’ for writing a sentence (or longer piece of poetry or prose) in which the lengths of successive words represent the digits of the number π, and his wonderful website is a treasure trove of Pilish literature, from plays, poems (called piems in Pilish), accidental Pilish and more. Keith's book, Not a Wake, is written entirely in Pilish from the first 10,000 digits π (the only book to have ever been written in Pilish: see this article from Alex Bellos for more). This, for example, from Keith in 1986, gives π to 356 places:
For a time I stood pondering on circle sizes. The large computer mainframe quietly processed all of its assembly code. Inside my entire hope lay for figuring out an elusive expansion. Value: pi. Decimals expected soon. I nervously entered a format procedure. The mainframe processed the request. Error. I, again entering it, carefully retyped. This iteration gave zero error printouts in all — success. Intently I waited. Soon, roused by thoughts within me, appeared narrative mnemonics relating digits to verbiage! The idea appeared to exist but only in abbreviated fashion — little phrases typically. Pressing on I then resolved, deciding firmly about a sum of decimals to use — likely around four hundred, presuming the computer code soon halted! Pondering these ideas, words appealed to me. But a problem of zeros did exist. Pondering more, solution subsequently appeared. Zero suggests a punctuation element. Very novel! My thoughts were culminated. No periods, I concluded. All residual marks of punctuation = zeros. First digit expansion answer then came before me. On examining some problems unhappily arose. That imbecilic bug! The printout I possessed showed four nine as foremost decimals. Manifestly troubling. Totally every number looked wrong. Repairing the bug took much effort. A pi mnemonic with letters truly seemed good. Counting of all the letters probably should suffice. Reaching for a record would be helpful. Consequently, I continued, expecting a good final answer from computer. First number slowly displayed on the the flat screen — 3. Good. Trailing digits apparently were right also. Now my memory scheme must probably be implementable. The technique was chosen, elegant in scheme: by self reference a tale mnemonically helpful was ensured. An able title suddenly existed — “Circle Digits”. Taking pen I began. Words emanated uneasily. I desired more synonyms. Speedily I found my (alongside me) Thesaurus. Rogets is probably an essential in doing this, instantly I decided. I wrote and erased more. The Rogets clearly assisted immensely. My story proceeded (how lovely!) faultlessly. The end, above all, would soon joyfully overtake. So, this memory helper story is incontestably complete. Soon I will locate publisher. There a narrative will I trust immediately appear, producing fame. THE END.
Writing in Pilish, i.e., writing a sentence (or longer piece of poetry or prose) in which the lengths of successive words represent the digits of the number π, has been popular since the early 1900s. There are of course rules needed, not least for example with respect to how you deal with the digit zero, or how we deal with long runs of successive digits, like 111, or 11112211? This page from Michael Keith explains how to write in basic or standard Pilish.
Michael Keith also created the piku, a piem based on the Japanese Haiku (see see here). In a piku in addition to successive words representing the digits of the number π, the number of syllables in each line is 3, 14, and 15, reflecting the digits of π. Here's a wonderful example from Keith:
It’s a moon,
A wheel revolving on golden earth, and lotus blossoms.Mountains embrace windmills, and it all reflects this number, pi.
Accidental Pilish is where a text is found to have be written in Pilish. The longest example from Shakespeare, found by Mike Keith is from Act II, Scene IV of King Henry the Fourth Part One:
FALSTAFF: By the mass, lad, thou sayest true; it is like we shall have good trading that way. But tell me, Hal, art not thou horrible afeard? Thou being heir apparent, could the world pick thee out three such enemies again as that fiend Douglas, that spirit Percy, and that devil Glendower? Art thou not horribly afraid? Doth not thy blood thrill at it?
PRINCE: Not a whit, i’ faith. I lack some of thy instinct.
It's also fun to reimagine texts in Pilish, for example the title of literary classics, songs, films, etc.
'Pi', by Kate Bush
This song, 'Pi', by Kate Bush from her 2005 album 'Aerial', is an ode to a 'sweet and gentle and sensitive man, with an obsessive nature and deep fascination for numbers, and a complete infatuation for the calculation of pi'. (You can view the lyrics to the song here.) Throughout the song Kate sings this number:
3.14159265358979323846264338327950288419716939937510582319749445923078164062862088214808651328230664709384460955058223
You will note, however, that whilst the 55th digit of π is 0, Kate sings 3 and then 1, before getting back on track and singing the next 24 digits correctly. She then, however, completely misses out the next 22 digits of π before singing the next 37 digits correctly.
3.14159265358979323846264338327950288419716939937510582097494459230781640628620899862803482534211706798214808651328230664709384460955058223
I wonder if she sings the song live?
'Repeated Sequence,' by Martin Krzywinski
This piece, by Martin Krzywinski (see the π inspired Art section for more from Martin), with Norweigian band Flunk, Gregory Coles, and others is an entrancing musical performance of π digits. It was Krzywinski's project to celebrate PiDay 2023, (see his page here for more fascinating information about how the piece was made). It is a 1 hour Repeated Sequence, where the digits of π up to the Feynman Point (769 digits) are read out in English, Norewigian and Turkish. (Norwegian: 0 nul, 1 én, 2 to, 3 tre, 4 fire, 5 fem, 6 seks, 7 sju, 8 åtte, 9 ni. Turkish: 0 sıfır, 1 bir, 2 iki, 3 üç, 4 dört, 5 beş, 6 altı, 7 yedi, 8 sekiz, 9 dokuz.) And if you'd like to 'chill out to 10 hours of π' here is the team's 10 hour version, where the first 10,000 digits are read out.
'What pi sounds like,' by Michale Blake
On PiDay 2011, Michael Blake released this song, 'What pi sounds like', which translated π first few dozen digits into musical notes. However, jazz musician Lars Erickson, in the words of a NewScientist article, 'thought Blake’s work sounded suspiciously similar to his own 1992 piece “Pi Symphony,” also based on the digits of pi, which [was] registered with the US copyright office.'
'Pi Symphony,' by Lars Erickson
Erickson brought a lawsuit for copyright infringement, but on PiDay 2012, US district court judge Michael H. Simon dismissed the claim. In dissmissing the claim, the judge said, 'Pi is a non-copyrightable fact, and the transcription of pi to music is a non-copyrightable idea... The resulting pattern of notes is an expression that merges with the non-copyrightable idea of putting pi to music.'
'Pi Rap' by MC Hammersmith
Here is a fun Pilish PiDay rap to remember the first 100 digits of π, from MC Hammersmith, the multi-award winning hip hop comedian, where the number of letters in each word of the rap correspond to the digits of π.
A selection of π related puns and jokes. I have indicated the writer of the joke where known, or source. If you know a good and accessible π joke or pun to add to the collection, please do tweet me (@mathsedideas).
My pin is the last four digits of π.
― HT @DrTrapezio
Q: How are mathematicians like the air force?
A: They both use pi lots.
Q: Why is 6 afraid of π?
A: I don't know; it’s an irrational fear.
Approximately 3.14% of sailors are pi-rates.
― Adapted from @ThePunnyWorld
Without pi our opinions would just be onions.
Pie is on sale at 3.1 four two.
Q: How many stars did the movie critic give to Life of Pi?
A: 3.14159
Never argue with π, he's irrational.
Q: What is a mathematician's favourite snake?
A: A Pi-thon.
Q: What so you get if you divide 22 sheep into 7 pens?
A: A Shepherd's Pi (approximately).
― HT @DadJokeMan
Q: What do you get when you divide the Sun's circumference by its diameter?
A: Pi in the sky.
Q: What do you get if you divide the circumference of a pumpkin by its diameter?
A: A pumpkin pi.
Doctor: How bad is your pain on a scale of 1-10?
Me: π
Doctor: ?
Me: It's not too bad but it goes on forever.
Eating too much cake is the sin of gluttony.
However, eating too much pie is OK because the Sin of Pi is 0.
― HT @ThePunnyWorld
Q: Why shouldn’t you eat too much pi?
A: You’ll end up with a big circumference.
Never start talking to π. He'll go on forever.
Q: What is the volume of a pizza with radius z and thickness a?
A: Pizza (or Pi-zza).
What do you mean π\({r^2}\)? Pie are round.
Q: What did the students say about their advance geometry test?
A: It was easy as pi.
Q: What musical instruments do mathematicians play?
A: The pi-ano.
i was arguing with π. i said, "Get real," said π. "You're being irrational."
I hate all these π jokes. They go on forever.
Our fascination with and desire to better understand the transcendence of π (and indeed other such constants) has inspired much incredible and innovative art. Mathematician and logician John Venn, for example, in his efforts to understand and explain randomness, was the 'first person to visualize the random nature of pi’s decimal digits,' as shared by Alex Bellos in this article. Venn, in the words of Lukas Verburgt in this article, thus 'presented the first graph of a random walk and anticipated the notion of a fractal.' Venn produced the image below, from The Logic of Chance (1888, p118), to graphically illustrate, in the words of Verburgt, 'the randomness of the digits of pi by using them to generate a path described by a point. His starting point is to work with a compass with eight points (numbered 0–7) to direct the movement at each step on a square lattice: 0 is north, 1 is northeast, and so on.' In Venn's own words, 'the result seems to me to furnish a very fair graphical indication of randomness.' (Jeffrey Thompson updated Venn's random walk slightly to incorporate the digits 0-9; you can view the result here.)
Amongst his amazing body of work, Martin Krzywinski produces stunning data visualizations related to π. His π-day page dedicated to π inspired visualizations is a wonderful treasure trove of beautiful images to get lost in, with accompanying explanations about how they were generated. The example below is a poster Krzywinski produced for π-day 2014. The image, he explains, is the 'Frequency distribution of digits in Pi for the first 4,988 digits of Pi in groupings of 4. This subset contains the triplets for each digit, the last being 888 at digit 4,985. The layout is on an Archimedean spiral. For each grouping the number of times a digit was seen is proportional to the width of the annulus.' You can buy this artwork here,
Think Maths have collated ideas for school π art projects that can be viewed here. And Pink Stripey Socks sugges a range of PiDay activities, including for preschoolers, such as making a PiDay Bracelet. Creating a Pi Skyline is also a popular activity. This page from What Do We Do All Day? goes into detail about how, and gives examples. The image below is an example of a Pi Skyline for the first 70 digits of π.
Audio
- Archimedes, BBC In Our Time (45m)
- As Simple as Pi, BBC 'Simon Singh's Numbers' (14m)
- Legislating Pi, Futility Closet (30m)
- Marcus Du Sautoy on Pi, Oxford University The Secrets of Mathematics (13m 28s)
- Pi, from BBC In Our Time (45m)
- Pi, from BBC David Baddiel Tries to Understand (15m)
- The Problem of Infinite Pi(e), BBC The Curious Cases of Rutherford and Fry (36m)
Video
- Calculating Pi by weighing a circle, by Matt Parker (4m 13s)
- Calculating Pi with a Pendulum, by Matt Parker (4m 17s)
- Calculating Pi with real Pies, from Numberphile (3m 14s)
- Calculating Pi with Darts, by Physics Girl with Derek Muller (5m 47s)
- Data Visualisation The Beauty of Pi, from Numberphile (8m 23s)
- Generating π from 1000 random numbers, by Matt Parker (23m 59s)
- How Pi was nearly changed to 3.2, from Numberphile (4m 55s)
- Marcus Du Sautoy on Pi, Oxford University The Secrets of Mathematics (13m 28s)
- π tape, from Do Online Now Guys (10m 17s)
- Pi and Bouncing Balls, from Numberphile (13m 14s)
- Sounds of Pi, from Numberphile (6m 28s)
- Why Pi is Awesome, by TheOdd1sOut with Vi Hart (7m 47s)
Websites
- Dedicated PiDay site
- Find a string of numbers in pi
- Find a string of numbers in the 1st 2 billion digits of π
- Martin Krzywnski's PiDay Art page
- Pi Art Projects, from Think Maths
- Pi Day Activities for Preschoolers, from Pink Stripey Socks
- The Pi Day Recipe Book, from Math with Bad Drawings
- San Fransisco's 'Exploratorium' π-day page (the founders of π-day)
- three.onefouronefivenine.com, by Christian Lawson-Perfect
- Wolfram demonstrations linked to π (Applet)
Articles
- Formula for pi discovered in hydrogen atoms, Science Alert
- Is there any importance for pi being transcendental, Quora
- Pi transformed into incredible art – in pictures, Alex Bellos, The Guardian
- Shakespeare, Jane Austen and the poet laureate of pi, Alex Bellos, The Guardian
- The Transcendence of π, Niven 1939
- The Transcendence of π, Steve Mayer 2006
[1] Or Half Tau Day! See the Tau manifesto by Michael Hartl to make your own mind up.
3Blue1Brown. (2018, March 2). Why is pi here? And why is it squared? A geometric answer to the Basel problem [Video File]. Retrieved from https://youtu.be/d-o3eB9sfls
3Blue1Brown. (2018, March 2). Why is pi here? And why is it squared? A geometric answer to the Basel problem [Video File]. Retrieved from https://youtu.be/d-o3eB9sfls
Akira Haraguchi. (n.d.). In Wikipedia. Retrieved from https://en.wikipedia.org/wiki/Akira_Haraguchi
BBC Newsnight. (2017, August 27). Autistic savant Daniel Tammet on ‘the language of numbers. Retrieved from https://youtu.be/j3Mecg8iuuE
Bellos, A. (2014, March 14). 'Pi Day: pi transformed into incredible art – in pictures,' The Guardian. Retrieved from https://www.theguardian.com/science/alexs-adventures-in-numberland/gallery/2014/mar/14/pi-day-pi-transformed-into-incredible-art-in-pictures
Bellos, A. (2015). 'He ate all the pi : Japanese man memorises π to 111,700 digits', The Guardian. Retrieved from https://www.theguardian.com/science/alexs-adventures-in-numberland/2015/mar/13/pi-day-2015-memory-memorisation-world-record-japanese-akira-haraguchi
Bellos, A. (2015). 'He ate all the pi : Japanese man memorises π to 111,700 digits', The Guardian. Retrieved from https://www.theguardian.com/science/alexs-adventures-in-numberland/2015/mar/13/pi-day-2015-memory-memorisation-world-record-japanese-akira-haraguchi
Blinder, S.M. (n.d.). "Viète's Nested Square Root Representation of Pi", Wolfram Demonstrations Project. Retrieved from http://demonstrations.wolfram.com/VietesNestedSquareRootRepresentationOfPi/
Bonch-Osmolovskaya, T. (2012). 'Art of π: Mathematical History and Literary Inspiration', Bridges 2012: Mathematics, Music, Art, Architecture, Culture, pp.79-84. Retrieved from https://archive.bridgesmathart.org/2012/bridges2012-79.pdf
Borwein, M., Borwein, P.B., and Bailey, D.H. (1989). 'Ramanujan, Modular Equations, and Approximations to Pi or How to Compute One Billion Digits of Pi,' The American Mathematical Monthly, Vol.96, No.3, pp.201–219. Retrieved from https://www.jstor.org/stable/2325206
Casselman, B. (2012, February). 'Archimedes on the Circumference and Area of a Circle', American Mathematical Society. Retrieved from http://www.ams.org/publicoutreach/feature-column/fc-2012-02
Cho, A. (2015, November 15). 'Famed number π found hidden in the hydrogen atom', Science. Retrieved from http://www.sciencemag.org/news/2015/11/famed-number-found-hidden-hydrogen-atom
Cipra, B. (206). 'Digits of Pi', What's Happening in the Mathematical Sciences. Vol. 6. American Mathematical Society. Retrieved from http://www.ams.org/publicoutreach/math-history/hap-6-pi.pdf
Flood, R. & Wilson, R. (n.d.). 'Jon Wallis'. University of Oxford, Mathematical Institute. Retrieved from https://www.maths.ox.ac.uk/system/files/media/John%20Wallis.pdf
Freiberger, M. (2016, August 6). 'The Basel Problem', Plus Magazine. Retrieved from https://plus.maths.org/content/basel-problem
Hafner, I. (2013, August 22). "Newton's Pi Approximation", Wolfram Demonstrations Project. Retrieved from http://demonstrations.wolfram.com/NewtonsPiApproximation/
Jones, W. (1706). Synopsis Palmariorum Matheseos: Or, A New Introduction to the Mathematics: Containing the Principles of Arithmetic and Geometry. London. Retrieved from https://archive.org/details/SynopsisPalmariorumMatheseosOrANewIntroductionToTheMathematics
Kate Bush. (n.d.). In Wikipedia. Retrieved from https://en.wikipedia.org/wiki/Kate_Bush
Jones, W. (1706). Synopsis Palmariorum Matheseos: Or, A New Introduction to the Mathematics: Containing the Principles of Arithmetic and Geometry. London. Retrieved from https://archive.org/details/SynopsisPalmariorumMatheseosOrANewIntroductionToTheMathematics
Kate Bush. (n.d.). In Wikipedia. Retrieved from https://en.wikipedia.org/wiki/Kate_Bush
Lewis, T. (2015, March 13). 'Pi, Anyone? The Secret to Memorizing Tens of Thousands of Digits', Live Science. Retrieved from https://www.livescience.com/50134-pi-day-memory-experts.html
MathWithoutBorders (2012, April 9). Finding Pi by Archimedes' Method [Video File]. Retrieved from https://youtu.be/_rJdkhlWZVQ
Neale, V. (n.d.). Sieve of Eratosthenes. NRICH. University of Cambridge. Retrieved from https://nrich.maths.org/7520
O'Connor, J.J. and Robertson, E.F. (2000, January). 'François Viète', School of Mathematics and Statistics, University of St Andrews. Retrieved from http://www-groups.dcs.st-and.ac.uk/history/Biographies/Viete.html
Pickover, C. (2008). Archimedes to Hawking: Laws of Science and the Great Minds Behind Them. Oxford: Oxford University Press. p111.
Popova, M. (2015). 'Brainman: Inside the Mind of an Autistic Savant: What the circumference of a circle has to do with on-demand polyglotism', Brain Pickings [Blog post]. Retrieved from https://www.brainpickings.org/2011/03/08/brainman-daniel-tammet-documentary/
Ramanujan Prime (n.d.). In Wikipedia. Retrieved from https://en.wikipedia.org/wiki/Ramanujan_prime
Popova, M. (2015). 'Brainman: Inside the Mind of an Autistic Savant: What the circumference of a circle has to do with on-demand polyglotism', Brain Pickings [Blog post]. Retrieved from https://www.brainpickings.org/2011/03/08/brainman-daniel-tammet-documentary/
Ramanujan, S. (1914). 'Modular Equations and Approximations to π,' The Quarterly Journal of Mathematics, 45, pp.350–372.
The RSA. (2013, January 23). How Maths Illuminates Our Lives - Daniel Tammet [Video File]. Retrieved from https://youtu.be/1gfFiReLA70
Sangwin, C.J. (2001, December 1). 'An infinite series of surprises', Plus Magazine. Retrieved from https://plus.maths.org/content/infinite-series-surprises
Schreiber, M. (n.d.). "Wallis Sieve Pi Approximation", Wolfram Demonstrations Project. Retrieved from http://demonstrations.wolfram.com/WallisSievePiApproximation/
Sergeyev, Y.D. (2012). 'Evaluating the exact infinitesimal values of area of Sierpinski's carpet and volume of Menger's sponge', Chaos, Solitons & Fractals, Vol. 42, pp 3042-3046. Retrieved from https://arxiv.org/abs/1203.3150
Staple, D. (2015). 'The combinatorial algorithm for computing pi(x)', Dalhouse University Faculty of Graduate Studies Online Theses. Retrieved from http://dalspace.library.dal.ca/handle/10222/60524
Summer House. (2012, June 9). Savant learns how to speak Icelandic in a week. Retrieved from https://youtu.be/_GXjPEkDfek
TeachPi.org (n.d.). Ludolph van Ceulen (1540 – 1610): Tireless hand-calculator who took pi to 35 digits & had them engraved on his tombstone. Retrieved from http://www.teachpi.org/stories/devoted-beyond-death/
Sangwin, C.J. (2001, December 1). 'An infinite series of surprises', Plus Magazine. Retrieved from https://plus.maths.org/content/infinite-series-surprises
Schreiber, M. (n.d.). "Wallis Sieve Pi Approximation", Wolfram Demonstrations Project. Retrieved from http://demonstrations.wolfram.com/WallisSievePiApproximation/
Sergeyev, Y.D. (2012). 'Evaluating the exact infinitesimal values of area of Sierpinski's carpet and volume of Menger's sponge', Chaos, Solitons & Fractals, Vol. 42, pp 3042-3046. Retrieved from https://arxiv.org/abs/1203.3150
Staple, D. (2015). 'The combinatorial algorithm for computing pi(x)', Dalhouse University Faculty of Graduate Studies Online Theses. Retrieved from http://dalspace.library.dal.ca/handle/10222/60524
Summer House. (2012, June 9). Savant learns how to speak Icelandic in a week. Retrieved from https://youtu.be/_GXjPEkDfek
TeachPi.org (n.d.). Ludolph van Ceulen (1540 – 1610): Tireless hand-calculator who took pi to 35 digits & had them engraved on his tombstone. Retrieved from http://www.teachpi.org/stories/devoted-beyond-death/
Weisstein, E.W. "Prime Counting Function." From MathWorld--A Wolfram Web Resource. Retrieved from http://mathworld.wolfram.com/PrimeCountingFunction.html
Weisstein, E.W. "Sierpiński Arrowhead Curve." From MathWorld--A Wolfram Web Resource. Retrieved from http://mathworld.wolfram.com/SierpinskiArrowheadCurve.html
Weisstein, E.W. "Sierpiński Carpet." From MathWorld--A Wolfram Web Resource. Retrieved from http://mathworld.wolfram.com/SierpinskiCarpet.html
Weisstein, E.W. "Sierpiński Curve." From MathWorld--A Wolfram Web Resource. Retrieved from http://mathworld.wolfram.com/SierpinskiCurve.html
Weisstein, E.W. "Sierpiński Sieve." From MathWorld--A Wolfram Web Resource. Retrieved from http://mathworld.wolfram.com/SierpinskiSieve.html